Multi-Digit Multiplication Strategies and Introducing Gizmos

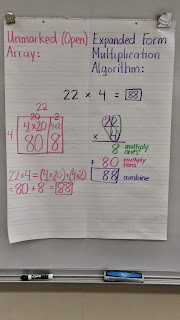
With a short week, we're taking some time to practice multi-digit multiplication.  We're transitioning from using unmarked (open) arrays modeling partial products to using partial products with the expanded form multiplication algorithm.  We'll be working on 2 digit x 1 digit and 2 digit x 2 digit situations this week.  Over the next few weeks, we'll use the expanded form multiplication algorithm for 3 digit x 1 digit and 4 digit x 1 digit situations.  After Winter Break, we'll learn the U.S. standard multiplication algorithm for these situations.  So, until then, please be patient as your student develops greater number sense and is laying a solid foundation for better understanding and correctly using the U.S. standard multiplication algorithm.  :-)

Here's a video that shows how we have used an unmarked (open) array to model partial products.  Please ignore the last part about the standard algorithm for now.  :-)

This video will give you an idea of how to use the expanded form multiplication algorithm.  Honestly, I think the notation in this video is cluttery, so in class we think aloud most of the steps and just write the partial products , then combine them.  We've been using colored pencils to reinforce this when we practice:

We're also reviewing division with remainders this week.  One curriculum resource we're using is Gizmos.  Gizmos are online math and science simulations for third grade students and older.  Your student's Gizmo login information is in the front of her/his planner.  I added some math and science Gizmos from past units in case you would like your student to complete them for remediation or extra practice (or just for fun!).  By clicking on the "lesson info" link once you're logged in, you can print vocabulary sheets and student exploration packets like we use in class.  I recommend spending no longer than 30 minutes per session as most Gizmos contain multiple activities.  We're currently working on No Alien Left Behind (division and division with remainders).